

If you need to do this by hand, then you'd just plug in more #y#'s and solve for #x#. The equation of a line is typically written as ymx+b where m is the slope and b is the y-intercept. Vertex point: () Further point: () Computing a quadratic function out of three points Enter three points. If you are required to find more coordinates, the easiest way to get them is to just plug the equation into your calculator, and get more points. Calculate a quadratic function given the vertex point Enter the vertex point and another point on the graph. that best fits our data points (x 1, y 1). The aim of quadratic regression is to find an equation in the form: y a + bx + cx². Lastly, you just need to draw a curve through all these three points, and you are done! This is what it would look like: Let x be the explanatory variable and y the response variable. Hence our intercepts would be the points #(7,0)# and #(5,0)# Now let's find the zeroes of our sample equation: These will give you additional points to complete your curve.
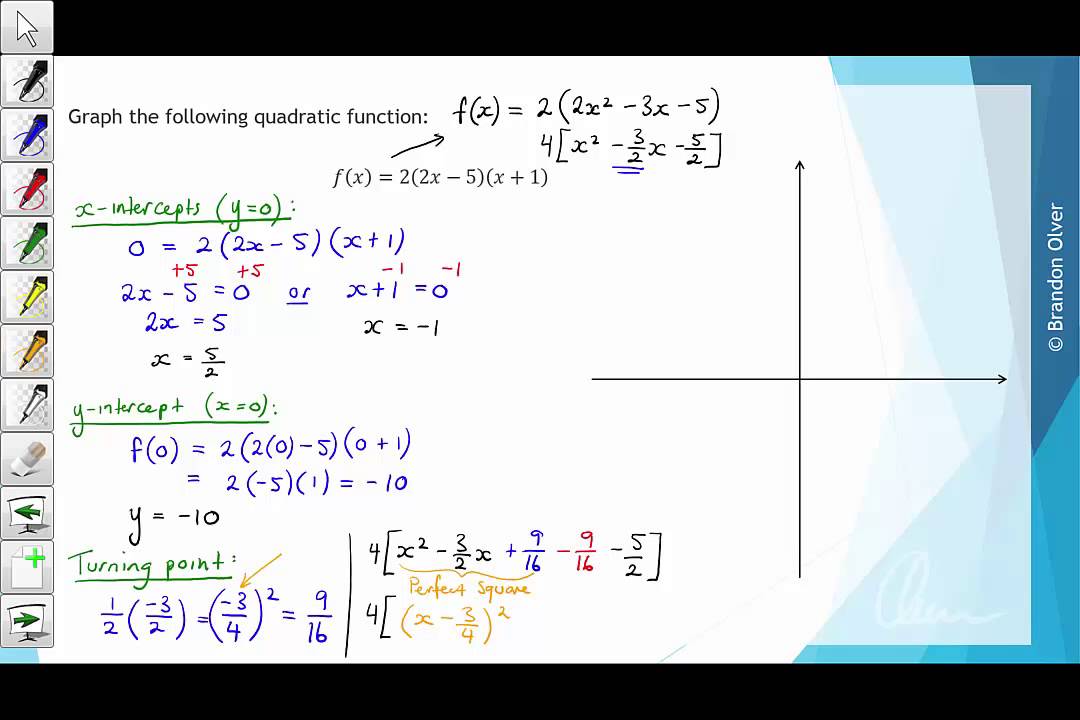
If you plug in 0 for #y# in the equation above, and solve for #x#, then you will fnd the coordinates of the two intercepts. Thirdly, you need to find the intercepts of the graph. This will help you get a good picture of what your graph will look like.Ĭonsidering our sample equation, #a = -2#, therefore it opens down. If it is positive, then it opens upwards. If your #a# value in the above equation is negative, then the equation opens downwards. Secondly, you need to find the direction of the graph. This calculator will find either the equation of the parabola from the given parameters or the vertex, focus, directrix, axis of symmetry, latus rectum. Here #h = 6# and #k = 2#, therefore the vertex would be #(6,2)# Identify and interpret roots, intercepts and turning points of quadratic. It is either the highest or lowest point on your graph, and it is the first thing you need to graph.įor example, consider the equation #y = -2(x-6)^2+2# An online tool to draw, display and investigate graphs of many different kinds. In the equation #y=a(x-h)^2+k#, the point #(h,k)# is your vertex. To find x - coordinate of the vertex we use formula: So, we substitute in for and in for to get To find y - coordinate plug in into the original equation: So, the vertex of the parabola is STEP 2: Find the y-intercept. This is derived through (wait for it) vertex form. Example 1: Sketch the graph of the quadratic function Solution: In this case we have and STEP 1: Find the vertex. Note that the quadratic formula actually has many real-world applications, such as calculating areas, projectile trajectories, and speed, among others.When you graph a quadratic, there are a couple of things you need to consider that will make your life easier.įirstly, you need to find the vertex. This is demonstrated by the graph provided below. Furthermore, the quadratic formula also provides the axis of symmetry of the parabola. Example 1: Sketch the graph of the quadratic function Solution: In this case we have and STEP 1: Find the vertex. Equation of a line given two points first calculator finds the line equation in slope-intercept form, that is, yax+b. The x values found through the quadratic formula are roots of the quadratic equation that represent the x values where any parabola crosses the x-axis. Recall that the ± exists as a function of computing a square root, making both positive and negative roots solutions of the quadratic equation. That parabola can intersect the x -axis in zero, one or two points: Related quadratic equation has no real solutions, meaning that the discriminant is less than. Below is the quadratic formula, as well as its derivation.įrom this point, it is possible to complete the square using the relationship that:Ĭontinuing the derivation using this relationship: Only the use of the quadratic formula, as well as the basics of completing the square, will be discussed here (since the derivation of the formula involves completing the square). A quadratic equation can be solved in multiple ways, including factoring, using the quadratic formula, completing the square, or graphing.
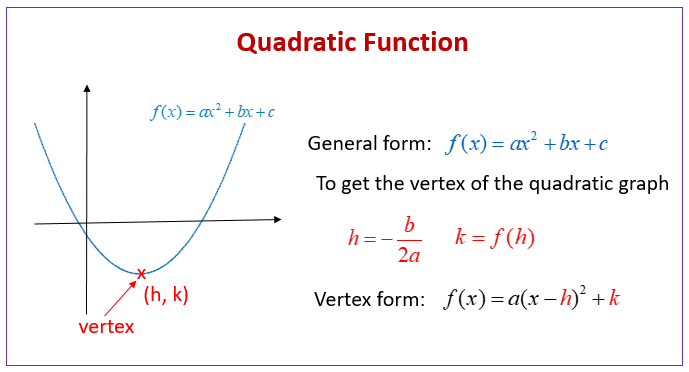
For example, a cannot be 0, or the equation would be linear rather than quadratic. The numerals a, b, and c are coefficients of the equation, and they represent known numbers. Where x is an unknown, a is referred to as the quadratic coefficient, b the linear coefficient, and c the constant. This formula, x b±b24ac 2a x b ± b 2 4 a c 2 a, determines the one or two solutions to any given quadratic. In algebra, a quadratic equation is any polynomial equation of the second degree with the following form: One common method of solving quadratic equations involves expanding the equation into the form ax2 +bx+c 0 a x 2 + b x + c 0 and substituting the a a, b b and c c coefficients into a formula known as the quadratic formula. Fractional values such as 3/4 can be used.


 0 kommentar(er)
0 kommentar(er)
